Information
In the moon emporium we provide a number of amazing and safe attractions that will have everyone having a blast. Each one is custom designed for the moon and its unique gravity. We have also insured to create sealed pods for one or two people to sit inside so that you can have the time of your life and not fear suffocation.
Let us explain some of our favourite attractions and show you that we have created both the safest and most thrilling experience. Below we have detailed diagrams, calculations and explanations that will help you understand the achievement that we have given you. The untrained average human can handle a max of 5g (Sands 2021), so we have taken the care that all of our rides do not exceed this limit, will still employing maximum thrill.

The Cannon Shot
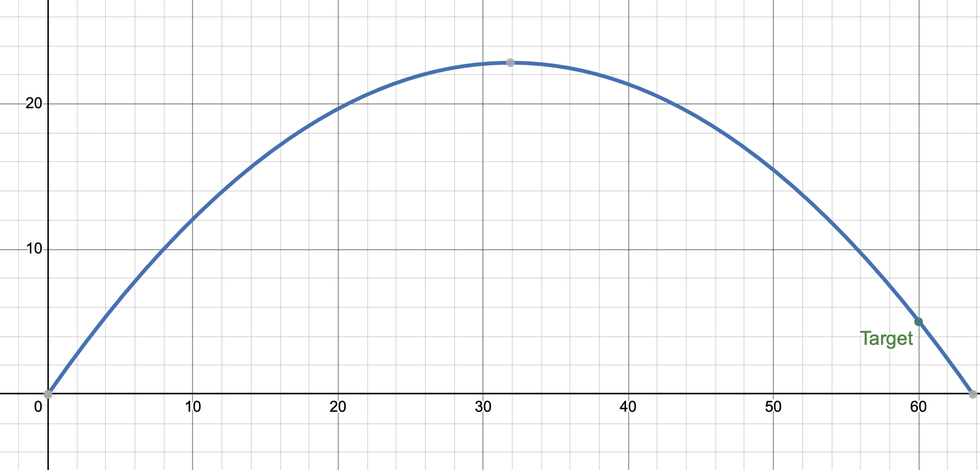
The Cannon Shot is a fun to use attraction for all ages. It starts with in a special control bubble with a strong glass wall that is able to view the cannon the attraction is named after. Your job is to aim the cannon so that when it shoot the shot will hit a target 60m away. Some rules that we have implemented to ensure that everyone has a fair go is that the shot can only stay in the air for a maximum of 10 seconds. This is to ensure that one shot does not take too long and everyone can have a turn. The other restriction is that the the cannon has 80 degrees of freedom so that there is no situation where the cannon is aimed back at you.

As an example we can calculate the exact angle and initial velocity needed to hit the target right at the 10 second mark, the maximum amount of time. This is simple to do by considering the x and y components of the trajectory as the I and j components of a vector equation. Subbing in the values and considering the influence of gravity in the y component means that we can solve the exact initial velocity and angle needs to hit the target at 10 seconds. This results in an angle of 55.1 degrees and an initial velocity of 10.5m/s. Lastly we have a general equation in order to calculate the initial velocity and angle if given either one. Note that the formatting for the math is based on Specialist Maths level of understanding on Vectors and Projectile Motion.

We can easily graph the trajectory of the projectile with the trajectory formula above and graphing tools such as demos. This shows us that the projectile will hit the target. What's great is that this is not the only way to hit the target, by changing the angle and strength of the cannon you might be able to hit the target even faster then what we have shown you.
The Cavatappi
Named after the pasta, this roller coaster is aimed at an older audience. It starts with you and one other person in a safely sealed pod so that you can have a great and safe experience. You then start with a slow climb up to a height of 100 meters. Then comes the main attractions were you travel down a downwards spiral. the spiral is made up of five different loops, the first four are the same with a radius of 5 meters. the fifth one however has a radius of 10 meters. While this might seem scary we can prove that it is perfectly safe to enjoy. The diagram below shows different points of the spiral in each loop, each loop is 15 meters higher than the previous one. This is useful as we can use the formulas for gravitational and kinetics energy to calculate the velocities at each point with a maximum load of 750 kg.
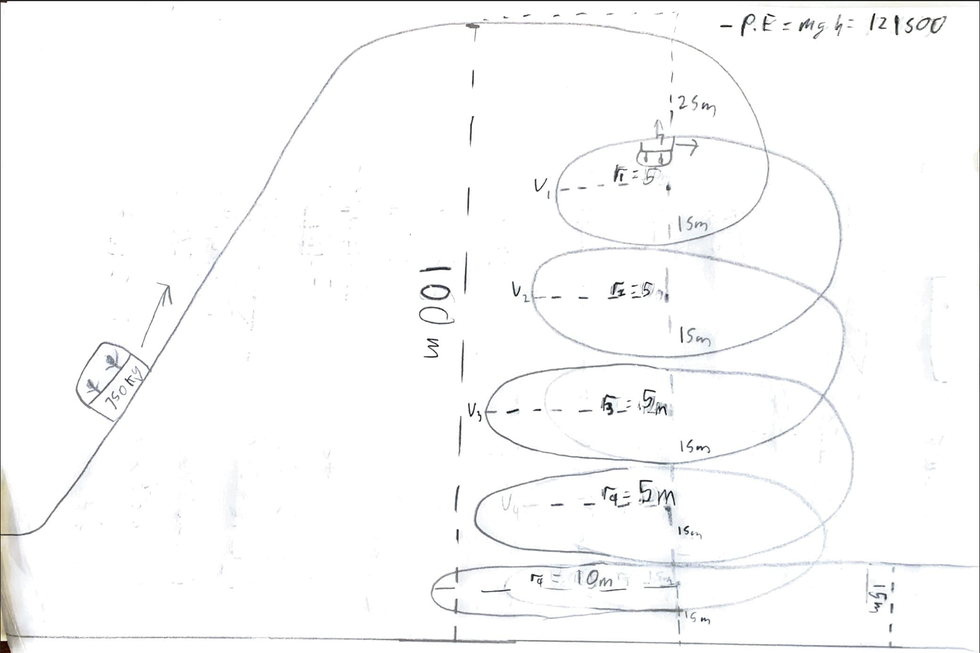
Since the highest point and start of the spiral is 100m we can calculate that Gravitational potential energy at the top is 121500J of energy. We can also calculate the kinetic energy at the last loop by minusing the remaining potential energy, giving us 103275J of Kinetic energy, since we know the highest of the other points then we can repeat the process for them. Since this is circular motion then we are able to calculate the acceleration at each point by squaring the velocity and dividing by the radius. Finally we can calculate the g force by adding the moon's gravity and dividing earth's gravity. This means that we get a final set of calculations showing us that the ride peaks at 4.8g. It also shows us why we had increased the radius on the final loop, 4.8g is quite close to the limit that normal people can handle so if we had not then the peak amount of g's felt would be higher then it is now and possibly be a risk for your health.

If we were to repeat the same calculations for the absolute minimum weight (the 600kg pod by itself) then we would find that nothing would change when it comes to the velocities, therefore the acceleration and g-force won't change since there equations are not influenced by mass. We can then finally calculate the net force which always points towards the centre of the radius.

With the net force we can then calculate the Normal force experienced at each point with a free body diagram. an example of this is shown below.


The Lunar Spinner
The Lunar Spinner, the Final Attraction that we will talk about is a Star flyer (2025, Funtime Manufacturers) inspired attraction that places you inside of a pod connected by chains by yourself, lifts you up and spins you around similar to a rotor. The attractions will then build up speed until the period of time needed for one rotation is ten seconds. The pod is 200kg and can hold up to 120 kg, so at max speed as shown in the diagram the pod will rise up by 45 degrees. At rest the radius from the pod to the centre is 40 meters, however this will change when the attraction reaches max speed. Since we know it maxes out at 45 degrees then we can calculate the max radius from the centre will basic trig functions.

This will result in a total radius of 89.5 meters, we can also show the new height at 50.5 meters. With the radius then we can use circular motion equations for velocity, since we know that the period is equal to 10 seconds at its fastest. This means that the max velocity is equal to 56.2m/s. Using the same acceleration formula as earlier, then we can calculate the acceleration and g-force to be 35.3m/s^2 and 3.8g. This is ideal as this sits in our range of 2 to 5g.

Finally we have a free body diagram to display the how gravity, net Force and Normal look at any point in time. As such we can calculate the magnitude of the normal to be 11307 Newtons.
Create Your Own Website With Webador